The Time I Dropped Out of Math Class in the Fourth Grade
I confused social convention for human brilliance.
This is probably overly confessional for a newsletter, but a memory crashed back into my head last week that’s extremely pedagogically formative for me and also relevant to our current moment where teachers are working with students with a really wide range of understanding from the last year of remote teaching.
I was homeschooled from kindergarten through eighth grade with a five-hour break in the fourth grade where I begged to be enrolled in the neighborhood public school and then begged to be unenrolled.
My mom and I visited my new teacher after school. Everything checked out. The classroom looked like a classroom, not a dining room, and I was kind of excited about that part maybe. The teacher gave me the day’s homework to take home and complete.
I got home and I got very stuck and then I didn’t go back to school.
I still remember the assignment, decades later. We were supposed to cut out plastic straws and tape them to a sheet in the shape of scalene, isosceles, and equilateral triangles.
These were names I had never seen before, through no one’s fault except the arbitrary ordering of mathematical ideas in two different curricula, but that was that.
What is (mathematical) truth but a lie agreed upon?
So much of mathematics rests on the foundation of social convention. Why is the horizontal axis labeled “x” instead of “m.” Why do we plot the horizontal coordinate first. Why do we evaluate arithmetic operations in the order we do.
You can’t take the square root of a negative number until you can. The angles of a triangle always add up to 180° until they don’t. Polygons always have an integer number of sides until they don’t.
It seems very easy, as a teacher, to ask questions that ensure only students who understand a social convention look mathematically smart—like wearing glasses that filter out every color but one.
It seems very easy, as a student, to try to answer those questions, run aground on the social convention, and assume that I am not mathematically smart.
Some #educatorgoals then:
Help students understand that their question “Why is it that way? Why couldn’t it have been another way” is an important and necessary one and has been, in fact, a primary engine for the advancement of the field of mathematics throughout history.
Help students understand other conventions were possible. “Yeah, we could have decided the first coordinate was the vertical coordinate. That would have been just fine. We had to decide on something though and you and I weren’t in the room.”
Ask the questions that gave rise to the need for a social convention.
We developed a coordinate system to describe location precisely, so consider giving students the opportunity to try to describe location precisely without one.
We developed names for triangles because certain triangles exhibited certain interesting characteristics we wanted to group together. So consider asking students to look at a bunch of triangles and group them in ways they find interesting.
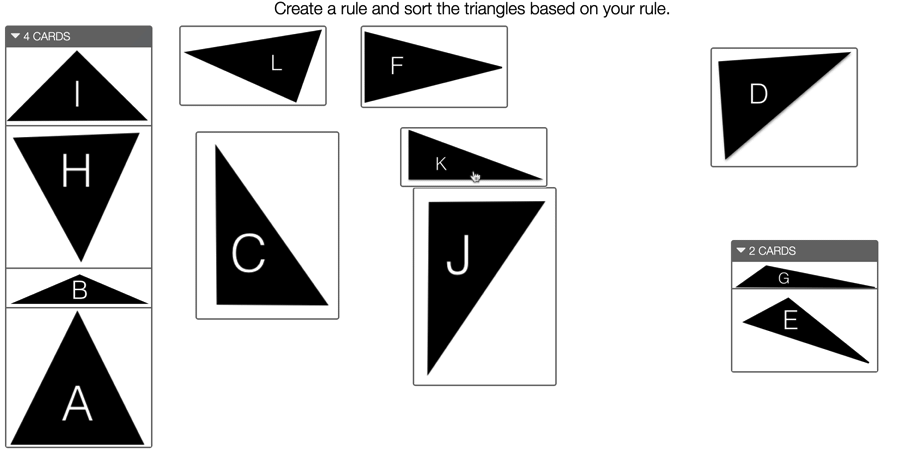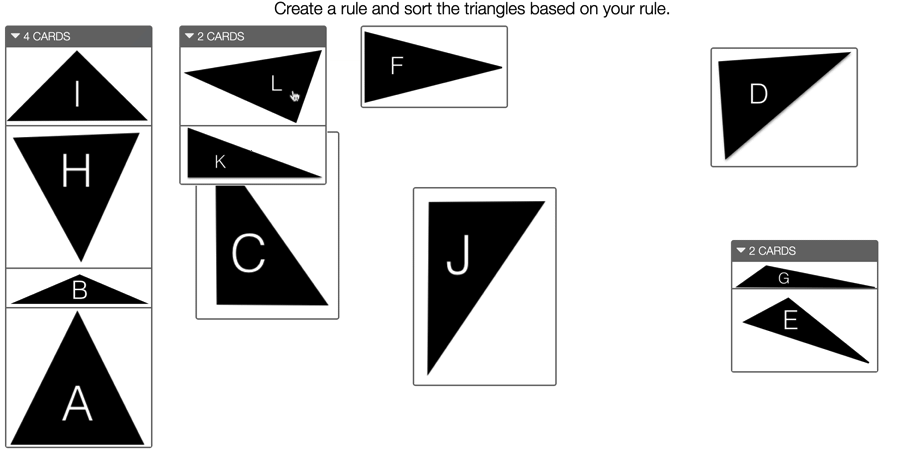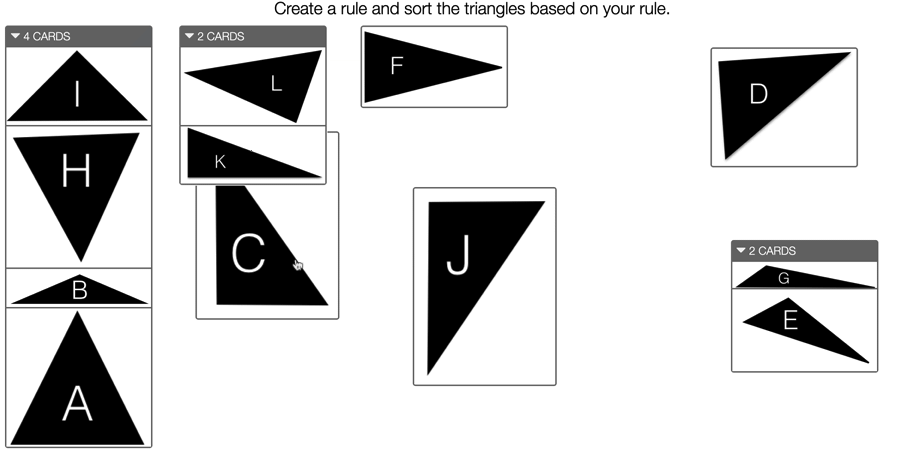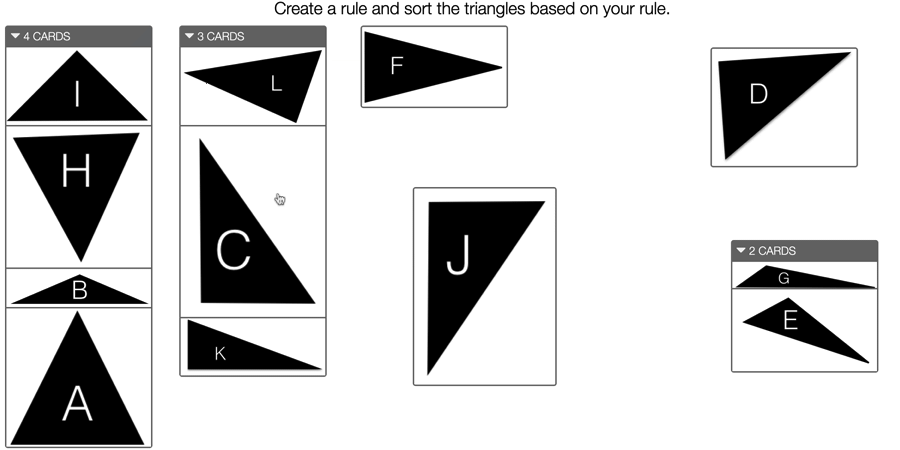
We created social conventions to serve our needs, not the other way around. We created social conventions—scalene, isosceles, etc.—to facilitate our brilliance. But social conventions aren’t a substitute for human brilliance, nor should we ever let social conventions undermine a student’s appreciation for human brilliance or their sense of themselves as brilliant.
➕ Updates
Great recommendations from Mylène DiPenta.

🎁 What Else?
Is a circle a regular polygon? Look at this beautiful mind out there picking fights with parents at the Minnesota State Fair.
Sarah Carter’s free start-of-the-year resources are getting downloaded left and right. Also—love this idea about creating emergency sub plans as Desmos activities.
Ontario’s Jason To is on a podcast talking about his board’s work de-tracking their math classes.
Rose Lam hosts a great conversation about how much emphasis to give your syllabus at the start of school.
If my adventures learning math through homeschooling interest you, here’s an old post where I describe learning from a proto Khan Academy in the eighth grade.
📬 Mailbag
In my last newsletter, I asked you to share, “How did your journey with math and numbers begin? Where does your enthusiasm, your passion come from?” Some fantastic responses follow.
Linda L.
My HS Calculus teacher (1971-1972 school year) turned math class on its head for me. Each day he posed an interesting problem and put us to work in groups to grapple with it, followed by a lively whole-class discussion. I absolutely loved his class - first time I wasn't bored stiff in a math class. I decided to major in math and later to become a math teacher, because I fell in love with math that year and wanted to do for other kids what he did for me. Almost 50 years later and newly retired, I hope I had some success at that.
50 years! The impact education has on macro-level factors like GDP and life expectancy is questionable, but it’s undeniable that a single teacher in a single year can have a massive impact on the course of a kid’s life.
Sarah N. loves math precisely because of the opportunities it gave her to challenge social convention.
As a young child I would "sneak" my mom's puzzle books, erase her answers, and then redo the puzzles. I have always enjoyed a challenging puzzle, and I think that just flowed over into math. I'm fascinated by the idea of math concepts growing and changing. Such as the idea that we "can't" divide by zero... why not? It was once believed that we "can't" take the square root of a negative, yet we do! I like the creative and rebellious side of mathematics.
Ashley T describes #familygoals for me.
My mom was (and still is) a math teacher and so math was a natural part of my daily routine (we actually used to do mental math at the dinner table before eating together) so relationships with numbers began early. But as I grew, I actually began to struggle at random times in math throughout my high school and my college career as a math major. Along the way there were always specific teachers encouraging me, supporting and growing me. Realizing this, and how important persistence was to a math mindset, my passion for both teaching and social emotional learning grew. As a teacher that is what I founded our classroom culture on and now it has greatly impacted my work as a math specialist. My mom, sister (also a math teacher), and I have a group text message that is almost like our family PLC where we chat math and teaching and I just love how we can all be so enthusiastic and bond together over what we do.
Mark V’s love for math and numbers evolved and hopped across careers.
I remember in 4th grade crying in the classroom when I raced to take my answers to the teacher to get them checked and having to return, more than once, to redo them. So much for timed math tasks. She didn't do that much after that. I remember competing against John Wambaugh and Barry Moat to see who could do better at the work given us or on tests. We were good at it. But, competing to get some kind of prize, such as a grade, isn't what gave me a love for math. I think the first real eye opener came in college when in Elementary Analysis we derived the Fournier Series, and for the first time, I made the connection how the strain you could measure at an oil well at the surface related to what happens downhole at the pump. Basically, it is differential equations in action. I was an engineer 20 years, then came to my passion, teaching.




I love this post, so don't take this critique the wrong way (in fact, I might be wrong):
"Why do we evaluate arithmetic operations in the order we do?"
I don't believe this is a social convention. I believe the order of operations follows from our place-value system.
2,355 = 2(10^3)+3(10^2)+5(10^1)+5(10^0)
PEMDAS is what makes the value of a number. At least that's what I've been telling my students.