If you ask people at the highest levels of education technology, “What is digital technology’s greatest gift to education?” their answers often circle themes of scalability, affordability, speed, and evaluation.
The greatest gift of digital technology, to hear them tell it, is that it lets us take certain educational experiences, distribute them at low cost and instantaneous speed on an unprecedented scale, and then evaluate the results.
That is all a gift, certainly. The key point of tension between me and much of my industry is that I only want to scale experiences that exceed a particular threshold of quality. I do not care to scale experiences that fall below that threshold no matter how cheaply or quickly we can do it.
And the great gift of digital technology is, in my mind, that it fundamentally changes the kinds of experiences students can have with mathematics.
Here I could point you to the experiences students have in our curriculum powering submarines, creating pinwheels, sampling animal populations, racing turtles, balancing hangars, completing mini golf holes, building robots, etc—transformative experiences without any clear analog in the print world. But it’s worth pointing out here that a small amount of digital technology can make a transformative difference with even very old, analog experiences.
Low Floor, Moving Ceiling Tasks
Don Seward has the earliest mention I can find of what is now a very common phrase in math education1:
All good tasks should be, by design or fortunate accident, "low threshold, high ceiling". Low threshold so that nearly all people can make a start and high ceiling so that there's always some higher order avenue to work towards.
He attaches an example:
In these tasks, I am glad the floor is low (smaller squares) and the floor is high (larger squares). As a designer of digital tasks, I ask myself:
Why must the ceiling be in view right now?
What does it do to the novice mathematician to see, right now, the height of the ceiling?
For students who are motivated to reach the ceiling as quickly as possible, are they getting as much value as they can out of each change in elevation from the floor to ceiling?
As a first pass, digital technology allows us to say, “Okay can we just talk about the 3x3 square first?”
Digital technology lets us wonder, “What if we didn’t even tell students what the task was about just yet? What if we just put students in a place to get curious about the task and find its edges?”
Pixels are cheap and paper is expensive, a fact which makes these transformations quite costly in the print world (nearly impossible) and quite affordable in the digital world.
What I notice about routines like Contemplate then Calculate, where Liz Clark-Garvey flashes a brief glimpse of a shape and takes noticings from around the room, and many others like it, is that they aren’t actually low floor, high ceiling tasks. The floor is low but the ceiling isn’t actually yet in view. The ceiling would only distract us from all there is to enjoy at lower elevations of the task. Digital technology lets us move the ceiling. Digital technology lets us linger in mathematics a little longer, lets us experience it in different, interesting ways using nothing more powerful than slide software.
We should prove ourselves faithful to high ideals about students, learning, and math and only then enjoy the gifts of scale, speed, and efficiency.
Odds & Ends
I have taken a “wait and see” stance towards augmented and virtual reality in education. Currently, the transformational value relative to cost x nausea seems … kind of mid? Yet I cannot deny this AR example from chemistry caught my eye. (Note, however, all of the chem teachers in the comments saying, “this isn’t actually how it works.”)
Generative AI is a hammer and no one knows what is and isn’t a nail. Excellent piece which gets at the core question about genAI in education. If genAI is a hammer, is education the process of building a home or washing the dishes? In one case, we just need the hammer to get better, hallucinate less, etc. In the other case, the hammer is the wrong tool for the job and no amount of improvement will change that fact.
I don’t often post jobs here but Director of Core 6-12 Math Product at Amplify is an extremely important position to me. We need someone here who can represent strong ideals about digital technology products and strong ideals about mathematics education.
My 2015 article “Missing the Promise of Mathematical Modeling” is getting the re-release treatment from NCTM this month. Free access.
Thanks to Ralph Pantozzi, Peg Smith, and others for their archival services here.





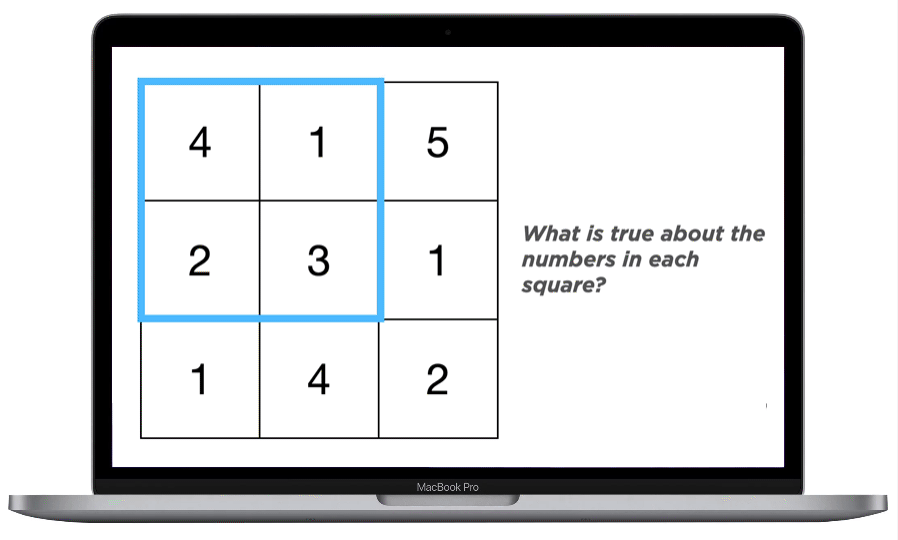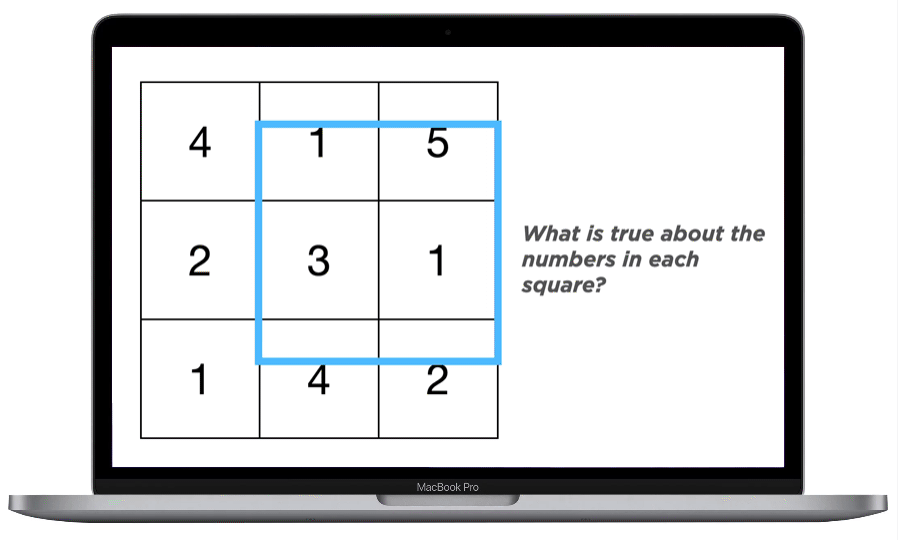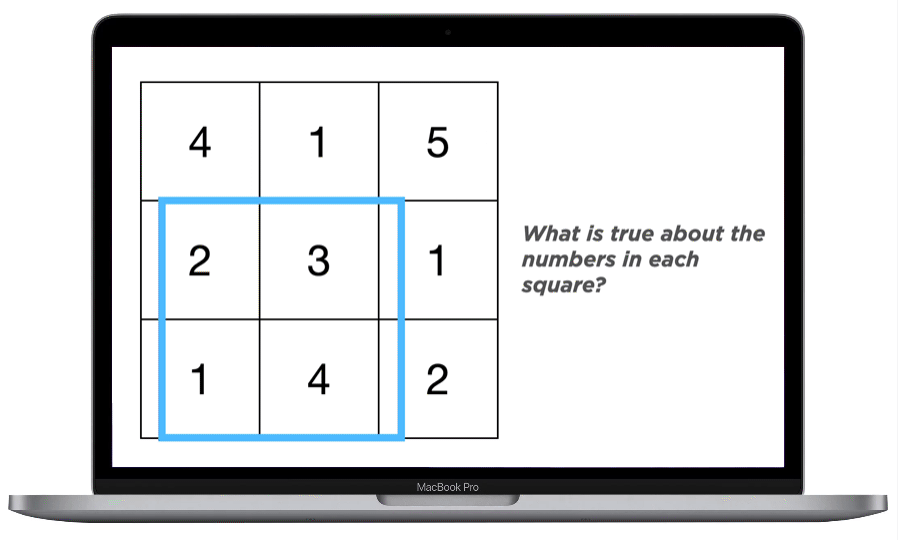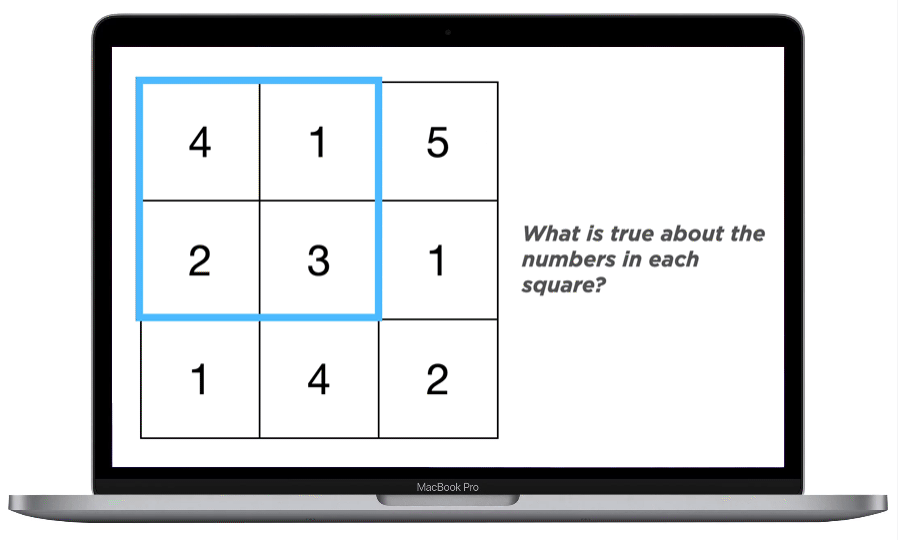


One of the things I like about using technology tools in elementary math is the interactive nature of the activities. Math labs using analog manipulatives are great, but costly and extraordinarily time consuming in set up and execution.
Low threshold-high ceiling comes from Seymour Papert many decades ago.